Role of Sample Size - Precision
 The
confidence interval represents the precision with which we are able to
report the effect size, and the larger the sample, the more precise the
estimate. As a practical matter, sample size is often the dominant factor
in determining the precision.
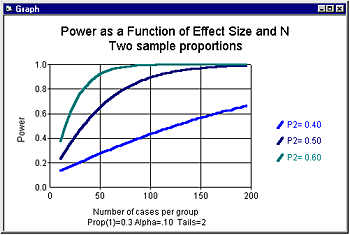
Figure 3 shows precision for a rate difference as a function of sample
size. This figure is based on the same rates used in the Power analysis
(30% vs. 50%). With N=50 per group the effect would be reported as 20
points with 95% confidence interval of plus/minus some 19 points (01 to
39 points). With N=100 per group the effect would be reported as 20 points
with 95% confidence interval of plus/minus some 13 points (7 to 33). With
N=200 per group the effect would be reported as 20 points with 95% confidence
interval of plus/minus some 9 points (11 to 29).
Note: For
studies that involve two groups precision is maximized when the subjects
are divided evenly between the two groups (this statement applies to the
procedures included in this program). When the number of cases in the
two groups is uneven the "effective N" for computing precision
falls much closer to the smaller sample size than the larger one.
Previous | Next
| 
